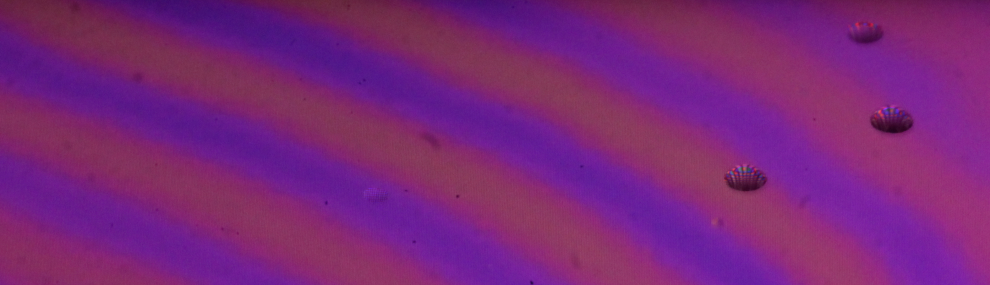
Liquid crystal theories are general mathematical descriptions of continua with some partial translational and rotational order. As such, they capture essential elements of patterns formed in various continuum systems. One example are wrinkles formed in a thin elastic sheet that is lying on a soft substrate, e.g. skin on flesh, when subject to an external forcing or as a result of geometric incompatibility. We study these systems from a smectic liquid crystal perspective, in order to make sense of the rich zoo of observed patterns. We develop methods to accurately predict, efficiently simulate, and universally design wrinkling patterns over many length scales.
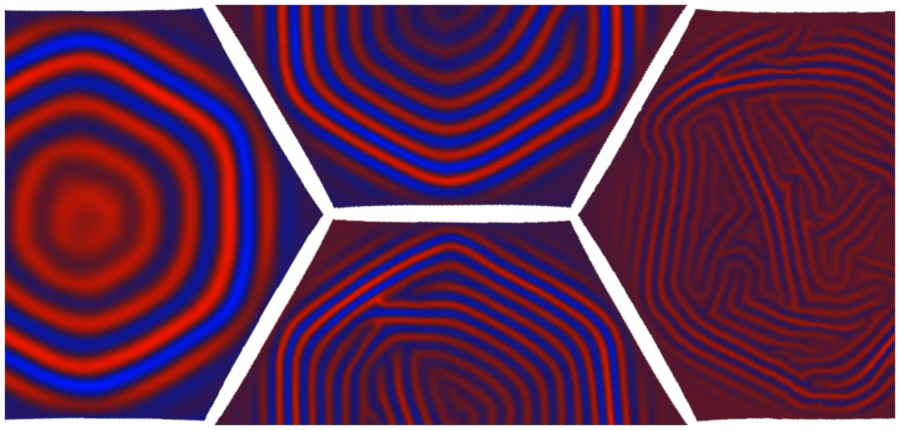
Wrinkling in a Floating Shell (source)